Patterns in Mathematics Class 6 Solutions Questions and Answers

1.1 What is Mathematics? Figure it Out (Page No. 2)

Question 1.
Can you think of other examples where mathematics helps us in our everyday lives?
Solution:
Math is very useful in our daily life. It help us to do many things like:

• Shopping and budgeting
• Navigation and travel
• Science and technology, etc. (Answer may vary)

Question 2.
How has mathematics helped propel humanity forward? (You might think of examples involving: carrying out scientific experiments; running our economy and democracy; building bridges, houses or other complex structures; making TVs, mobile phones, computers, bicycles, trains, cars, planes, calendars, clocks, etc.)
Solution:
Math is essential to science and technology, because it has real-world application in addition to helping us understand the world like help in creating more effective medical treatments. Mathematics algorithms and image processing methods are utilized in diagnostic imaging to reconstruct, evaluate and interpret the medical images received from X-Rays, CT scans, MRI and Ultrasounds, etc. (Answer may vary)

1.2 Patterns in Numbers Figure it Out (Page No. 3)

Question 1.
Can you recognize the pattern in each of the sequences in Table 1?

Solution:
Yes,

Patterns	Recognization of patterns
1, 1, 1, 1, 1, 1, 1,… (All l’s)	Sequence of all 1 ’s
1, 2, 3, 4, 5, 6, 7, … (Counting numbers)	A sequence of consecutive counting numbers starting from 1
1, 3, 5, 7, 9, 11, 13, … (Odd numbers)	A sequence of consecutive odd numbers starting from 1
2, 4, 6, 8, 10, 12, 14, … (Even numbers)	A sequence of consecutive even numbers starting from 2
1, 3, 6, 10, 15, 21, 28, … (Triangular numbers)	In the sequence, each term is the sum of first n consecutive counting numbers
1,4, 9, 16, 25,36, 49, … (Squares)	In the sequence, each term is the product of counting number by itself starting from 1
1, 8, 27, 64, 125, 216, … (Cubes)	In the sequence, each term is the product of counting number by itself thrice starting from 1
1, 2, 3, 5, 8, 13, 21, … (Virahanka numbers)	In the sequence, each term (starting from third term) is the sum of previous two terms

 

Question 2.
Rewrite each sequence of Table 1 in your notebook, along with the next three numbers in each sequence! After each sequence, write in your own words what is the rule for forming the numbers in the sequence.

Solution:

1, 1, 1, 1, 1, 1, 1, 1,1,1,1	Sequence of all 1 ’s
1, 2, 3, 4, 5, 6, 7, 8, 9, 10	A sequence of consecutive counting numbers starting from 1, adding 1 to the previous term to get the next term, as 1, 1 + 1 = 2, 2 + 1 = 3, 3 + 1 = 4, …
1,3, 5, 7, 9, 11, 13, 15, 17, 19	A sequence of consecutive odd numbers starting from 1, adding 2 to the previous term to get the next term, as 1, 1 + 2 = 3, 3 + 2 = 5, 5 + 2 = 7, 7 + 2 = 9, …
2, 4, 6, 8, 10, 12, 14, 16, 18, 20	A sequence of consecutive even numbers starting from 2, adding 2 to the previous term to get the next term, as 2, 2 + 2 = 4, 4 + 2 = 6, 6 + 2 = 8, 8 + 2= 10, …
1, 3, 6, 10, 15, 21, 28, 36, 45, 55	In the sequence, each term is the sum of first n consecutive counting numbers, as 1 = 1; 1 + 2 = 3; 1 + 2 + 3 = 6, 1 + 2 + 3 + 4 = 10; 1 + 2 + 3 + 4 + 5 = 15; 1 + 2 + 3 + 4 + 5 + 6 = 21; …
1, 4, 9, 16, 25, 36, 49, 64, 81, 100	In the sequence, each term is the product of counting number by itself starting from 1, as 1 × 1 = 1, 2 × 2 = 4, 3 × 3 = 9, 4 × 4 = 16, 5 × 5 = 25, …
1, 8, 27, 64, 125, 216, 343, 512, 729	In the sequence, each term is the product of counting number by itself thrice starting from 1, as 1 × 1 × 1 = 1, 2 × 2 × 2 = 8, 3 × 3 × 3 = 27, 4 × 4 × 4 = 64, 5 × 5 × 5 = 125, 6 × 6 × 6 = 216,…
1,2,3,5,8,13, 21,34, 55, 89	In the sequence, each term (starting from third term) is the sum of previous two terms, as 1, 2, 1 + 2 = 3, 2 + 3 = 5, 3 + 5 = 8, 5 + 8 = 13, 8 + 13 = 21, …
1, 2, 4, 8, 16, 32, 64, 128, 256, 512	In the sequence, next term is the double of previous term, as 1, 1 × 2 = 2, 2 × 2 = 4, 4 × 2 = 8, 8 × 2 = 16, 16 × 2 = 32,…
1,3, 9, 27,81, 243,729, 2187, 6561, 19683	In the sequence, ne×t term is the thrice of previous term, as 1, 1 × 3 = 3, 3 × 3 = 9, 9 × 3 = 27, 27 × 3 = 81, 81 × 3 = 243,…

1.3 Visualising Number Sequences Figure it Out (Page No. 5-6)

Question 1.
Copy the pictorial representations of the number sequences in Table 2 in your notebook, and draw the next picture for each sequence!

Solution:

Question 2.
Why are 1,3,6,10,15,… called triangular numbers? Why are 1, 4, 9, 16, 25, … called square numbers or squares? Why are 1, 8, 27, 64, 125, … called cubes?
Solution:

• The numbers 1, 3, 6, 10, 15, … are called triangular numbers because they can be represented by triangular arrangements of dots.
• The numbers 1,4,9,16,25,… are called square numbers or squares because they can be represented by square arrangements of dots.
• The numbers 1, 8, 27, 64, 125, … are called cubes, because they can be arranged in the form of cubes of unit blocks.

Question 3.
You will have noticed that 36 is both a triangular number and a square number! That is, 36 dots can be arranged perfectly both in a triangle and in a square. Make pictures in your notebook illustrating this!
This shows that the same number can be represented differently, and play different roles, depending on the context. Try representing some other numbers pictorially in different ways!
Solution:
36 as a triangular number
or

36 as a square number

In the same way, number 9 can be represented in different ways as,

Similarly, number 10 can be represented as a rectangle and a triangle by arranging dots, as

There are many more numbers that can be represented in different ways.

Question 4.
What would you call the following sequence of numbers?

That’s right, they are called hexagonal numbers! Draw these in your notebook. What is the next number in the sequence?
Solution:
∵ 1,7, 19, 37 are hexagonal numbers as they follow a pattern. As
1
1 + 6 = 7
7 + 12 = 19
19 + 18 = 37
37 + 24 = 61
So, the next hexagonal number in the sequence is 61.
It can represented as

Question 5.
Can you think of pictorial ways to visualise the sequence of Powers of 2? Powers of 3?
Here is one possible way of thinking about Powers of 2:

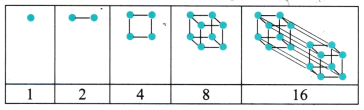
Solution:

Intext Questions

Question 1. (Page 7)
By drawing a similar picture, can you say what is the sum of the first 10 odd numbers?
Solution:

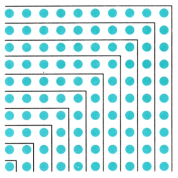
1 + 3+ 5+ 7 + 9 + 11 + 13 + 15 + 17 + 19 = 100

Question 2.
Now by imagining a similar picture, or by drawing it partially, as needed, can you say what is the sum of the first 100 odd numbers?
Solution:
Do it yourself by referring the above image.
We know that adding up consecutive odd numbers gives square numbers.
So, while adding up 100 odd number, we get a number which is square of 100.
1 + 3 + 5 + 7 + 9+ 11 + … + 197 + 199 = 10000

1.4 Relations Among Number Sequences Figure it Out (Page No. 8-9)

Question 1.
Can you find a similar pictorial explanation for why adding counting numbers up and down, i.e., 1, 1 + 2 + 1, 1 + 2 + 3 + 2 + 1, …, gives square numbers?
Solution:

 

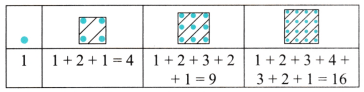
Question 2,.
By imagining a large version of your picture, or drawing it partially, as needed, can you see w hat will be the value of 1 + 2 + 3 + … + 99 + 100 + 99 + … + 3 + 2 + 1?
Solution:

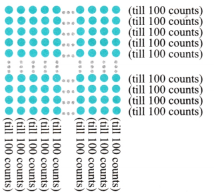
1 + 2 + 3 + … + 99 + 100 + 99 + … + 3 + 2 + 1 = 10000.

Question 3.
Which sequence do you get when you start to add the All 1 ’s sequence up? What sequence do you get when you add the All 1 ’s sequence up and down?
Solution:
When we add all 1 ’s sequence up, we get the counting numbers, as
1 = 1,
1 + 1 = 2,
1 + 1 + 1 = 3,
1 + 1 + 1 + 1 = 4,…
When we add all 1 ’s sequence up and down, we get counting numbers depends upon number of times 1 occurs.

Question 4.
Which sequence do you get when you start to add the Counting numbers up? Can you give a smaller pictorial explanation?
Solution:
We get the triangular numbers.
1, 1 + 2 = 3, 1 + 2 + 3 = 6, 1 + 2 + 3 + 4 = 10, 1 + 2 + 3 + 4 + 5 = 15,…
Pictorial representation:

Question 5.
What happens when you add up pairs of consecutive triangular numbers? That is, take 1+3,3 +.6, 6 + 10, 10 + 15, … ? Which sequence do you get? Why? Can you explain it with a picture?
Solution:
After adding up pairs of consecutive triangular numbers, we get square numbers. As
1 + 3 = 4, 3 + 6 = 9, 6 + 10 = 16, 10 + 15 = 25, 15 + 21 = 36,… Pictorial representation. As

Question 6.
What happens when you start to add up powers of 2 starting with 1, i.e., take 1, 1+2, 1+2 + 4, 1+2 + 4 + 8, … ? Now add 1 to each of these numbers — what numbers do you get? Why does this happen?
Solution:
On adding up powers of 2 starting with 1, as 1, 1 + 2 = 3, 1 + 2 + 4 = 7, 1 + 2 + 4 + 8 = 15, … we get a sequence like, 1 = 1, 1 × 2 + 1 = 3, 3 × 2 + 1 = 7, 7 × 2 + 1 = 15, 15 × 2 + 1 =31, …
Further adding up 1 to each number of the sequence obtained.
1 + 1 = 2, 3 + 1 = 4, 7 + 1 = 8, 15 + 1 = 16
Clearly, we get a number sequence of power of 2.
As, 2, 4, 8, 16, … .

 

Question 7.
What happens when you multiply the triangular numbers by 6 and add 1 ? Which sequence do you get? Can you explain it with a picture?
Solution:
The triangular numbers are 1, 3, 6, 10, 15, 21,…
After multiplying with 6 and adding 1, we get,
1 × 6 + 1 = 7, 3 × 6 + 1 = 19, 6 × 6 + 1 = 37, 10 × 6 + 1 = 61, 15 × 6+ 1 = 91,…
These are Hexagonal numbers.
Pictorial representation:

Question 8.
What happens when you start to add up hexagonal numbers, i.e., take 1, 1 + 7, 1 + 7 + 19, 1 + 7 + 19 + 37, … ? Which sequence do you get? Can you explain it using a picture of a cube?

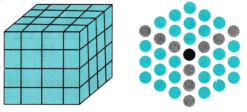
Solution:
Adding hexagonal numbers, we get
1, 1 + 7 = 8, 1 + 7 + 19 = 27, 1 + 7 + 19 + 37 = 64, …
Clearly, we get 1, 8, 27, 64, 125,…
It is the sequence of cubes.
Pictorial representation:

Question 9.
Find your own patterns or relations in and among the sequences in Table 1. Can you explain why they happen with a picture or otherwise?
Solution:
Do it yourself.

1.5 Patterns in Shapes Figure it Out (Page No. 11)

Question 1.
Can you recognise the pattern in each of the sequences in Table 3?

Solution:
Regular polygon: In the sequence, next polygon is obtained by increasing the number of sides by 1. Complete Graph: In the sequence, next shape is obtained by increasing the number of vertices by 1.
Stacked Squares: In the sequence, next bigger square is representing the square numbers.
Stacked Triangles: In the sequence, next bigger triangle is representing the sum of consecutive odd numbers starting from 1.
Koch Snowflake: In the sequence, each time each line segment ‘—’ is replaced by a speed bump

As one does this more and more times, the changes become tinier and tinier with very-very small line segments.

Question 2.
Try and redraw each sequence in Table 3 in your notebook. Can you draw the next shape in each sequence? Why or why not? After each sequence, describe in your own words what is the rule or pattern for forming the shapes in the sequence.
Solution:

 

1.6 Relation to Number Sequences Figure it Out (Page No. 11-12)

Question 1.
Count the number of sides in each shape in the sequence of Regular Polygons. Which number sequence do you get? What about the number of comers in each shape in the sequence of Regular Polygons? Do you get the same number sequence? Can you explain why this happens?
Solution:

The number sequence we get 3,4,5,6,7, 8,9,10, i.e., the counting numbers starting from 3, in both cases: number of sides and number of comers.
This happens as the number of comers depends upon the number of sides.

Question 2.
Count the number of lines in each shape in the sequence of Complete Graphs. Which number sequence do you get? Can you explain why?
Solution:

After counting the number of lines on each shape the sequence, we get
1, 3, 6, 10, 15, 21…,
It is the sequence of triangular numbers because each term of the sequence obtained is the sum of n consecutive numbers.

Question 3.
How many little squares are there in each shape of the sequence of Stacked Squares? Which number sequence does this give? Can you explain why?
Solution:

Clearly, we get a sequence of square numbers 1,4,9,16, 25, … Because we count the number of little squares in each shape and get each one is the product of counting number by itself.

Question 4.
How many little triangles are there in each shape of the sequence of Stacked Triangles? Which number sequence does this give? Can you explain why?
(Hint: In each shape in the sequence, how many triangles are there in each row?)
Solution:

Clearly, we get a sequence of square numbers, 1, 4, 9, 16, 25, … which are obtained by adding the number of small triangles in each row.
That is, 1, 1 + 3 = 4, 1 + 3 + 5 = 9, 1 + 3 + 5 + 7 = 16,…

 

Question 5.
To get from one shape to the next shape in the Koch Snowflake sequence, one replaces each line segment ‘—’ by a ‘speed bump’

As one does this more and more times, the changes become tinier and tinier with very- very small line segments. How many total line segments are there in each shape of the Koch Snowflake? What is the corresponding number sequence? (The answer is 3, 12, 48,…, i.e., 3 times Powers of 4;+his sequence is not shown in Table 1).
Solution:

By counting line segments, we get the number sequence: 3, 12, 48, 192, 768, …
In the sequence, each term is thrice of power of 4, as
3 × 1 = 3
3 × 4 = 12
3 × (4 × 4) = 48
3 × (4 × 4 × 4) = 192