Class 6 Maths Chapter 2 Lines and Angles Solutions

Lines and Angles Class 6 Solutions Questions and Answers

2.1 Point 2.2 Line Segment 2.3 Line 2.4 Ray Figure it Out (Page No. 15-17)

Question 1.

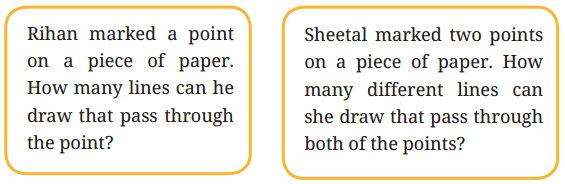
Can you help Rihan and Sheetal find their answers?
Solution:
Rihan can draw infinite number of lines that pass through the point.
Whereas Sheetal can draw only one line that passes through both of the points.

Question 2.
Name the line segments in the figure. Which of the five marked points are on exactly one of the line segments? Which are on two of the line segments?
Solution:
In the given figure, there are four line segments, i.e., \(\overline{\mathrm{LM}}, \overline{\mathrm{MP}}, \overline{\mathrm{PQ}}, \overline{\mathrm{QR}}\)
Points L and R are exactly on one of the line segments. Points M, P and Q on two of the line segments.

Question 3.
Name the rays shown in the figure below. Is T the starting point of each of these rays?

Solution:
Rays in figure are: \(\overrightarrow{\mathrm{TA}}, \overrightarrow{\mathrm{TN}}, \overrightarrow{\mathrm{TB}}\) and \(\overrightarrow{\mathrm{NB}}\)
No. [∵ T is not the starting point of NB]

Question 4.
Draw a rough figure and write labels appropriately to illustrate each of the following:
(a) OP and OQ meet at O.
Solution:

(b) XY and PQ intersect at point M.
Solution:

(c) Line l contains joints E and F but not point D.
Solution:

(d) Point P lies on AB.
Solution:

Question 5.
In the figure alongside, name:

(a) Five points
(b) A line
(c) Four rays
(d) Five line segments
Solution:
(a) Five points are: B, C, D, E and O
(b) A line is: DB (or BD)
(c) Four rays are: OB, OC, OD and OE
(d) Five line segments are: OB, OC, OD, DE and OE.

Question 6.
Here is a ray \(\overrightarrow{\mathrm{OA}}\). It starts at O and passes through the point A. It also passes through the point B.

(a) Can you also name it as \(\overrightarrow{\mathrm{OB}}\)? Why?
(b) Can we write \(\overrightarrow{\mathrm{OA}}\) as \(\overrightarrow{\mathrm{AO}}\) ? Why or why not?
Solution:
(a) Yes, rays \(\overrightarrow{\mathrm{OA}}\) and \(\overrightarrow{\mathrm{AO}}\) have same initial point and the same direction.
(b) No, rays \(\overrightarrow{\mathrm{AO}}\) has the initial point A, but in the given figure, the initial point is O. So, we can’t write \(\overrightarrow{\mathrm{OA}}\) as \(\overrightarrow{\mathrm{AO}}\).

 

2.5 Angle Figure it Out (Page No. 19-21)

Question 1.
Can you find the angles in the given pictures? Draw the rays forming any one of the angles and name the vertex of the angle.

Solution:
Yes, we can find the angles in the given pictures.

Question 2.
Draw and label an angle with arms ST and SR.
Solution:
We can draw an angle ∠RST of any measure with vertex S and arms \(\overrightarrow{\mathrm{ST}}\) and \(\overrightarrow{\mathrm{SR}}\).

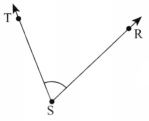
Question 3.
Explain why ∠APC cannot be labelled as ∠P.

Solution:
Using a single letter name like ∠P is insufficient in case of two or more angles having the same vertex. Here, ∠P can be considered for any of the three angles ∠APB, ∠BPC or ∠APC. So, here it is necessary to use three letters in specifying an angle.

Question 4.
Name the angles marked in the given figure.

Solution:
In the given figure, two angles have been marked with a common arm \(\overrightarrow{\mathrm{TR}}\). We can name these angles as ∠RTQ and ∠RTP or ∠PTR and ∠QTR.

Question 5.
Mark any three points on your paper that are not on one line. Label them A, B, C. Draw all possible lines going through pairs of these points. How many lines do you get? Name them. How many angles can you name using A, B, C? Write them down, and mark each of them with a curve.
Solution:
We can draw the figure as shown below. We get 3 lines in all. These are \(\overrightarrow{\mathrm{AB}}, \overrightarrow{\mathrm{BC}}\) and \(\overrightarrow{\mathrm{CA}}\).

We get 3 angles in all. These are ∠ABC, ∠BCA and ∠CAB.

Question 6.
Now mark any four points on your paper so that no three of them are on one line. Label them A, B, C, D. Draw all possible lines going through pairs of these points. How many lines do you get? Name them. How many angles can you name using A, B, C, D? Write them all down, and mark each of them with a curve.
Solution:
We can draw the figure as shown below. We get 6 lines in all. These are \(\overrightarrow{\mathrm{AB}}, \overrightarrow{\mathrm{BC}}, \overrightarrow{\mathrm{CD}}, \overrightarrow{\mathrm{DA}}, \overrightarrow{\mathrm{AC}}, \overrightarrow{\mathrm{BD}}\).

We get 12 angles in all. These are ∠ABC, ∠ABD, ∠GBD; ∠BCD, ∠BCA, ∠ACD; ∠CDA, ∠CDB, ∠BDA; ∠DAB, ∠DAC and ∠CAB.

 

2.6 Comparing Angles Figure it Out (Page No. 23)

Question 1.
Fold a rectangular sheet of paper, then draw a line along the fold created. Name and compare the angles formed between the fold and the sides of the paper. Make different angles by folding a rectangular sheet of paper and compare the angles. Which is the largest and smallest angle you made?

Solution:

Do it yourself. For your reference, ∠5 is the largest and ∠2 is the smallest among all the angles in the figure shown below.

Question 2.
In each case, determine which angle is greater and why.
(a) ∠AOB or ∠XOY
(b) ∠AOB or ∠XOB
(c) ∠XOB or ∠XOC

Discuss with your friends on how you decided which one is greater.
Solution:
(a) ∠AOB > ∠XOY because ∠XOY lies in the interior of ∠AOB.
(b) ∠AOB > ∠XOB because both angles have one arm OB common but uncommon arm OX of ∠XOB lies in the interior of ∠AOB.
(c) ∠XOB = ∠XOC because OB and OC both denote the same ray so, both angles have the common arms and the same vertex. That is, they superimpose to each other.

Question 3.
Which angle is greater: ∠XOY or ∠AOB? Give reasons.

Solution:
Using a single letter name like ∠P is insufficient in case of two or more angles having the same vertex. Here, ∠P can be considered for any of the three angles ∠APB, ∠BPC or ∠APC. So, here it is necessary to use three letters in specifying an angle.

2.7 Making Rotating Arms 2.8 Special Types of Angles Figure it Out (Page No. 29-31)

Question 1.
How many right angles do the windows of your classroom contain? Do you see other right angles in your classroom?
Solution:
Do it yourself.

Question 2.
Join A to other grid points in the figure by a straight line to get a straight angle. What are all the different ways of doing it?

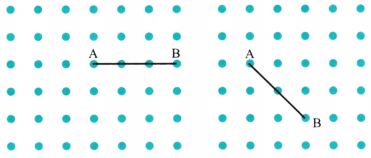
Solution:
We can produce BA beyond A to join other points of the grid. Thus we make a straight angle as shown in the figure below. Clearly, ∠CAB is a straight angle. There is only one way of doing it.

Question 3.
Now join A to other grid points in the figure by a straight line to get a right angle. What are all the different ways of doing it?

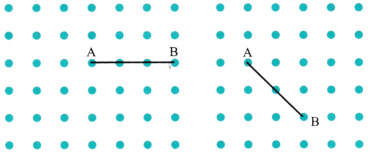
Solution:
We can produce BA beyond A to make a straight angle, then through A, draw the line DE, which is perpendicular to BC as shown in the figure below. Clearly, ∠DAB or ∠EAB is a right angle. There is only one way of doing it.

Question 4.
Get a slanting crease on the paper. Now, try to get another crease that is perpendicular to the slanting crease.
(a) Haw many right angles do you have now? Justify why the angles are exact right angles.
(b) Describe how you folded the paper so that any other person who doesn’t know the process can simply follow your description to get the right angle.
Solution:
We can fold the paper as shown in the figure.

(a) We get 4 right angles. Angle formed at any point and one side of a line is always a straight angle. The perpendicular drawn by the second crease through the vertex of a straight angle bisect it into two right angles.

(b) We first fold the paper to get a slanting crease and then fold again the paper in such a way that a part of the slanting crease falls on the other part of itself. Doing it carefully, we get another slanting line which is perpendicular to the first one. Thus, we get 4 right angles at the meeting point of the two lines as shown in the figure.

 

2.7 Making Rotating Arms 2.8 Special Types of Angles Figure it Out (Page No. 31-32)

Question 1.
Identify acute, right, obtuse and straight angles in the previous figures.
Solution:

Question 2.
Make a few acute angles and a few obtuse angles. Draw them in different orientations.
Solution:
Do it yourself.

Question 3.
Do you know what the words acute and obtuse mean? Acute means sharp and obtuse means blunt. Why do you think these words have been chosen?
Solution:
Acute mean ‘pointed’ and they are pointed at the vertex. They are less than a right angle comer. Obtuse Angles are ‘dull’ or ‘blunt’ and they form an angle that is not pointed, but duller. They are bigger than a right angle corner. Further, acute means narrow and obtuse means wider, that’s why these words have been chosen.

Question 4.
Find out the number of acute angles in each of the figures below.

What will be the next figure and how many acute angles will it have? Do you notice any pattern in the numbers?
Solution:
We find the number of acute angles in the given figures are:

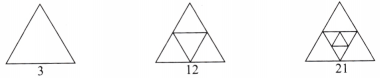
The next figure will be

So, the number pattern is 3, 3 + 9 = 12, 12 + 9 = 21, 21 + 9 = 30, …

InText Questions (Page 34)

Question 1.
The circle has been divided into 1, 2, 3, 4, 5, 6, 8, 9 10 and 12 parts below. What are the degree measures of the resulting angles? Write the degree measures down near the indicated angles.

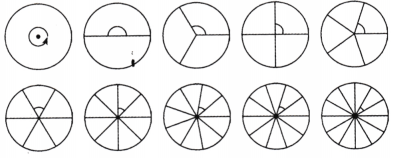
Solution:

2.9 Measuring Angles Figure it Out (Page No. 35)

Question 1.
Write the measures of the following angles:

(a) ∠KAL
Notice that the vertex of this angle coincides with the centre of the protractor. So the A number of units of 1 degree angle between KA and AL gives the measure of ∠KAL. By counting, we get ∠KAL = 30°.
Making use of the medium sized and large sized marks, is it possible to count the number of units in 5 s or 10s?
(b) ∠WAL
(c) ∠TAK
Solution:
(a) Yes it is possible to count the number of units in 5s or 10s because there are also marking for each 5° and 10° on the protractor and the arms \(\overrightarrow{\mathrm{AK}}\) and \(\overrightarrow{\mathrm{AL}}\) pass through the 10° markings.

(b) In the given figure, the arms \(\overrightarrow{\mathrm{AL}}\) and \(\overrightarrow{\mathrm{AW}}\) pass through the 10° markings. There are five 10° markings of scale where \(\overrightarrow{\mathrm{AL}}\) and \(\overrightarrow{\mathrm{AW}}\) pass. So, ∠WAL = 50°.

(c) In the given figure, the arms \(\overrightarrow{\mathrm{AK}}\) and \(\overrightarrow{\mathrm{AT}}\) pass through the 10° markings. There are twelve 10° markings of scale where \(\overrightarrow{\mathrm{AK}}\) and \(\overrightarrow{\mathrm{AT}}\) pass. So, ∠TAK = 120°.

 

InText Questions 

Think! (Pages 39-40)

Question 1.
In given figure, we have ∠AOB = ∠BOC = ∠COD = ∠DOE = ∠EOF = ∠FOG = ∠GOH = ∠HOI = ____.
Why?
Solution:
In the given figures 1 and 2, we observe that ∠AOB = ∠BOC = ∠COD = ∠DOE = ∠EOF = ∠FOG = ∠GOH = ∠HOI = 22.5°, because 180° is divided into 8 equal parts, i.e., \(\frac{180^{\circ}}{8}\) = 22.5°.

2.9 Measuring Angles Figure it Out (Page No. 40-43)

Question 1.
Find the degree measures of the following angles using your protractor.

Solution:

Question 2.
Find the degree measures of different angles in your classroom using your protractor.
Solution:
Do it yourself.

Question 3.
Find the degree measures for the angles given below. Check if your paper protractor can be used here!

Solution:
The degree measures for the angles shown below.

No, we cannot measures these angles by paper protractor.

Question 4.
How can you find the degree measure of the angle given below using a protractor?

Solution:
We can measure ∠1 = 100° using protractor and subtract it from 360° to find the measure of ∠2 = 260°.

Alternative: We can draw a ray opposite to one of the arms and split the angle into two parts – straight angle and an acute angle. The measure the acute angle (∠1 = 80°) using protractor and add it to 180°. It would be the measure of required angle (180° + 80° = 260°).

 

Question 5.
Measure and write the degree measures for each of the following angles:


Solution:
(a) 80°
(b) 120°
(c) 60°
(d) 130°
(e) 125°
(f) 62°

Question 6.
Find the degree measures of ∠BXE, ∠CXE, ∠AXB and ∠BXC.

Solution:
∠BXE = 115°, ∠CXE = 85°, ∠AXB = 65° and ∠BXC = 30°.

Question 7.
Find the degree measures of ∠PQR, ∠PQS and ∠PQT.

Solution:
∠PQR = 45°, ∠PQS = 100° and ∠PQT = 150°.

Question 8.
Make the paper craft as per the given instructions. Then, unfold and open the paper fully. Draw lines on the creases made and measure the angles formed.

Solution:
Do it yourself.

Question 9.
Measure all three angles of the triangle shown in the figure (a) given below and write the measures down near the respective angles. Now add up the three measures. What do you get?
Do the same for the triangles in the figure (b) and (c).
Try it for other triangles as well, and then make a conjecture for what happens in general! We will come back to why this happens in a later year.

Solution:

45°+ 65°+ 70°= 180°

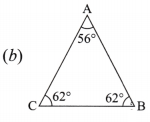
56° + 62°+ 62° = 180°

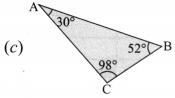
30° + 52° + 98° = 180°
In general, we observe that sum of all angles of a triangle is 180°.

 

2.9 Measuring Angles Figure it Out (Page No. 45-46)

Question 1.
Angles in a clock:
(a) The hands of a clock make different angles at different times. At 1 o’clock, the angle between the hands is 30°. Why?

Solution:
The clock dial has been divided into 12 equal parts. And the dial of clock represents angle of 360°.
So, angle measure of each part is 360° + 12 = 30°.
Thus, at 1 o’clock, the angle between the hands is 30°.

(b) What will be the angle at 2 o’clock? And at 4 o’clock? 6 o’clock?

Solution:
As from 12 to 2 we have 2 parts at 2 o’clock. So, the angle between the hands is 60°.
Similarly, between 12 and 4, we have four parts.
So, at 4 o’clock, the angle between the hands is 120°. Similarly, at 6 o’clock, the angle between the hands is 180°.

(c) Explore other angles made by the hands of a clock.
Solution:
(a)
Do it yourself.

Question 2.
The angle of a door:
Is it possible to express the amount by which a door is opened using an angle? What will be the vertex of the angle and what will be the arms of the angle?

Solution:
Yes, it is possible to express the amount by which a door is opened using an angle. The vertex of the angle will be hinge of the door and the arms of the angle will be wall and the door itself.

Question 3.
Vidya is enjoying her time on the swing. She notices that the greater the angle with which she starts the swinging, the greater is the speed she achieves on her swing. But where is the angle? Are you able to see any angle?

Solution:
Vidya’s original position is along’the vertical line. When she starts swinging the rope of the swing is along the slanting line which makes an angle with the imaginary vertical line. The speed depends upon this angle.

Question 4.
Here is a toy with slanting slabs attached to its sides; the greater the angles or slopes of the slabs, the faster the balls roll. Can angles be used to describe the slopes of the slabs? What are the arms of each angle? Which arm is visible and which is not?

Solution:
Yes, angles can be used to describe the slopes of the slabs. Horizontal imaginary line and slab are the arms of each angle. Here, slab arm is visible but horizontal line is not visible.

Question 5.
Observe the images below where there is an insect and its rotated version. Can angles be used to describe the amount of rotation? How? What will be the arms of the angle and the vertex?

Solution:
Observe the vertical and horizontal lines touching the insects. The rotation from vertical to horizontal position makes an angle. We can imagine the meeting point of the two lines as the vertex, and these two lines as arms of the angle as shown in the picture.

 

2.10 Drawing Angles Figure it Out (Page No. 49-50)

Question 1.
In the given figure below, list all the angles possible. Did you find them all? Now, guess the measures of all the angles. Then, measure the angles with a protractor. Record all your numbers in a table. See how close your guesses are to the actual measures.

Solution:

Name of Angles	Estimated measure of Angles	Actual measures of Angles
∠PAC	100°	107°
∠ACD	80°	72°
∠CDL	180°	180°
∠DLP	95°	97°
∠LPR	95°	98°
∠PLS	85°	82°
∠LSR	75°	78°
∠PRS	105°	102°
∠BRS	75°	79°

Question 2.
Use a protractor to draw angles having the following degree measures:
(a) 110°
(b) 40°
(c) 75°
(d) 112°
(e) 134°
Solution:
(a) We can construct an angle of measure 110° using a protractor step by step as discussed below.
Step I: Draw a ray \(\overrightarrow{\mathrm{AB}}\).

Step II: Place the centre of the protractor at A and the zero edge along \(\overrightarrow{\mathrm{AB}}\).

Step III: Start counting from zero near B. Mark a point P at 110° and join AP.

(c) We can construct an angle of measure 75° using a protractor step by step as discussed below.
Step I: Draw a ray \(\overrightarrow{\mathrm{AB}}\).

Step II: Place the centre of the protractor at A and the zero edge along \(\overrightarrow{\mathrm{AB}}\).

Step III: Start counting from zero near B. Mark a point P at 75°.

Step IV: Join AP.
Thus, ∠BAP = 75°.
(b) , (d) and (e). Do it yourself as done above.

Question 3.
Draw an angle whose degree measure is the same as the angle given below:

Also, write down the steps you followed to draw the angle.
Solution:
We can draw an angle equal to the given angle by following these steps:
Step I: Draw a ray \(\overrightarrow{\mathrm{AB}}\).

Step II: Measure the angle ZIHJ. It is 118°.
Step III: Place the centre of the protractor at A and the zero edge along \(\overrightarrow{\mathrm{AB}}\).
Step IV: Start counting from zero near B. Mark a point C at 118°.
Step V: Join AC.
Thus, ∠BAC = ∠IHJ.

 

2.11 Types of Angles and their Measures Figure it Out (Page No. 51-52)

Question 1.
In each of the below grids, join A to other grid points in the figure by a straight line to get:
Mark the intended angles with curves to specify the angles. One has been done for you.
(a) An acute angle

Solution:

(b) An obtuse angle

Solution:

(c) A reflex angle

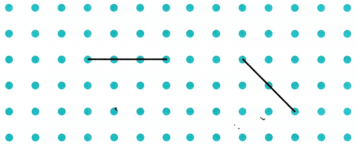
Solution:

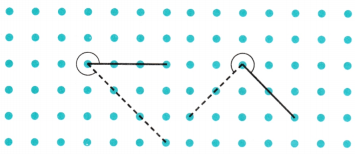
Question 2.
Use a protractor to find the measure of each angle. Then classify each angle as acute, obtuse, right, or reflex.
(a) ∠PTR
(b) ∠PTQ
(c) ∠PTW
(d) ∠WTP

Solution:
Using a protractor we fiipd the measure of each angle and then classify each angle as follows:
(a) ∠PTR = 31°, acute angle
(b) ∠PTQ = 60°, acute angle
(c) ∠PTW = 104°, obtuse angle
(d) ∠WTP = 360° – 104° = 256°, reflex angle.

 

InText Questions

Let’s Explore (Page 53)

Question 1.
In this figure, ∠TER = 80°.
What is the measure of ∠BET?
What is the measure of ∠SET?

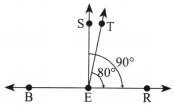
Solution:
Measure of ∠BET = Measure of ∠BER – Measure of ∠TER
= 180° – 80°
= 100°
Measure of ∠SET = Measure of ∠SER – Measure of ∠TER
= 90° – 80°
= 10°

2.11 Types of Angles and their Measures Figure it Out (Page No. 53-54)

Question 1.
Draw angles with the following degree measures:
(a) 140°
(b) 82°
(c) 195°
(d) 70°
(e) 35°
Solution:
Do it yourself.

Question 2.
Estimate the size of each angle and then measure it with a protractor:

Classify these angles as acute, right, obtuse or reflex angles.
Solution:
(a) 45°, acute angle
(b) 169°, obtuse angle
(c) 120°, obtuse angle
(d) 33°, acute angle
(e) 99°, obtuse angle
(f) 348°, reflex angle

 

Question 3.
Make any figure with three acute angles, one right angle and two obtuse angles.
Solution:
Sample figure

Question 4.
Draw the letter ‘M’ such that the angles on the sides are 40° each and the angle in the middle is 60°.
Solution:

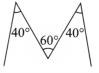
Question 5.
Draw the letter ‘Y’ such that the three angles formed are 150°, 60° and 150°.
Solution:

Question 6.
The Ashoka Chakra has 24 spokes. What is the degree measure of the angle between two spokes next to each other? What is the largest acute angle formed between two spokes?

Solution:
The Ashoka Chakra resemble with a circle. So its degree measure is 360°.
Dividing it into 24 equal parts by spokes, the measure of each part = 360° + 24= 15°
Thus, the degree measure of the angle between two spokes next to each other is 15°.
The largest acute angle formed between two spokes is 5 × 15° = 75°.

Question 7.
Puzzle: I am an acute angle. If you double my measure, you get an acute angle. If you triple my measure, you will get an acute angle again. If you quadruple (four times) my measure, you will get an acute angle yet again! But if you multiply my measure by 5, you will get an obtuse angle measure. What are the possibilities for my measure?
Solution:
As per given condition, we have 4 × acute angle < 90° and 5 × acute angle > 90°
⇒ acute angle < \(\frac{90^{\circ}}{4}\) = 22\(\frac{1}{2}^{\circ}\) and acute angle > \(\frac{90^{\circ}}{5}\) = 18°
So, possible measures of the acute angle would be 19°, 20°, 21° and 22° in whole numbers.